Difference between revisions of "GFDL:Lagrangian point"
m (→Stability: link tinkering) |
|||
| (27 intermediate revisions by 10 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{move2space}} | |
| + | {{Wikipedia}} | ||
| + | {{Cleanup}} | ||
| + | [[Image:Lagrange_B.jpg|thumb|right|330px|A contour plot of the effective [[potential]] (the [[Hill's Surfaces]]) of a two-body system (the [[Sun]] and [[Earth]] here), showing the five Lagrange points.]] | ||
| + | The '''Lagrangian points'''; also '''Lagrange point''', '''L-point''', or '''[[libration]] point'''), are the five positions in an [[orbit]]al configuration where a small object affected only by [[gravity]] can theoretically be stationary relative to two larger objects (such as a [[satellite]] with respect to the [[Earth]] and [[Moon]]). The Lagrange points mark positions where the combined gravitational pull of the two large masses provides precisely the [[centripetal]] force required to rotate with them. They are analogous to [[geosynchronous orbit]]s in that they allow an object to be in a "fixed" position in space rather than an orbit in which its relative position changes continuously. | ||
| − | + | A more precise but technical definition is that the Lagrangian points are the stationary solutions of the circular restricted [[N-body problem#Three-body problem|three-body problem]]. For example, given two massive bodies in circular [[orbit]]s around their common [[center of mass]], there are five positions in space where a third body, of comparatively negligible [[mass]], could be placed which would then maintain its position relative to the two massive bodies. As seen in a [[rotating reference frame]] with the same period as the two co-orbiting bodies, the [[gravitational field]]s of two massive bodies combined with the [[centrifugal force]] are in balance at the Lagrangian points, allowing the third body to be stationary with respect to the first two bodies. | |
| − | In | + | ==History and concepts== |
| + | In [[1772]], the famed Italian-French mathematician {{exd|Joseph-Louis Lagrange|Joseph-Louis Lagrange}} was working on the famous {{exd|Three Body Problem|three-body problem}} when he discovered an interesting quirk in the results. Originally, he had set out to discover a way to easily calculate the gravitational interaction between arbitrary numbers of bodies in a system, because [[Newtonian mechanics]] conclude that such a system results in the bodies orbiting [[chaos theory|chaotically]] until there is a collision, or a body is thrown out of the system so that [[Mechanical equilibrium|equilibrium]] can be achieved. The logic behind this conclusion is that a system with one body is trivial, as it is merely static relative to itself; a system with two bodies is very simple to solve for, as the bodies orbit around their common center of gravity. However, once more than two bodies are introduced, the mathematical calculations become very complicated. A situation arises where you would have to calculate every gravitational interaction between every object at every point along its trajectory. | ||
| − | [[ | + | Lagrange, however, wanted to make this simpler. He did so with a simple conclusion: ''The trajectory of an object is determined by finding a path that minimizes the [[action (physics)|action]] over time.'' This is found by subtracting the [[potential energy]] from the [[kinetic energy]]. With this way of thinking, Lagrange re-formulated the classical Newtonian mechanics to give rise to [[Lagrangian mechanics]]. With his new system of calculations, Lagrange’s work led him to [[hypothesis|hypothesize]] how a third body of negligible mass would orbit around two larger bodies which were already in a near-circular orbit. In a frame of reference that rotates with the larger bodies, he found five specific fixed points where the third body experiences zero net force as it follows the circular orbit of its host bodies (planets).<ref> |
| + | Joseph-Louis Lagrange - Oeuvres de Lagrange, 1867-1892; Gauthier-Villars. Tome 6, Chapitre II: Essai sur le problème des trois corps, 272-292, http://gallica.bnf.fr/Catalogue/noticesInd/FRBNF30719104.htm | ||
| + | </ref> These points were named “Lagrangian points” in Lagrange's honor. It took over a hundred years before his mathematical theory was observed with the discovery of the [[Trojan asteroids]] in the 1900s at the Lagrange points of the Sun-Jupiter system. | ||
| + | In the more general case of [[elliptical]] orbits, there are no longer stationary ''points'' in the same sense: it becomes more of a Lagrangian “area”. The Lagrangian points constructed at each point in time as in the circular case form stationary elliptical orbits which are [[Similarity (mathematics)#Geometry|similar]] to the orbits of the massive bodies. This is due to [[Newton's second law]] (<math>d\mathbf{p}/dt=\mathbf{F}</math>), where ''p = mv'' (''p'' the [[momentum]], ''m'' the mass, and ''v'' the velocity) is [[invariant (physics)|invariant]] if force and position are scaled by the same factor. A body at a Lagrangian point orbits with the same period as the two massive bodies in the circular case, implying that it has the same ratio of gravitational force to radial distance as they do. This fact is independent of the circularity of the orbits, and it implies that the elliptical orbits traced by the Lagrangian points are solutions of the [[equation of motion]] of the third body. | ||
| + | |||
| + | ==The Lagrangian points== | ||
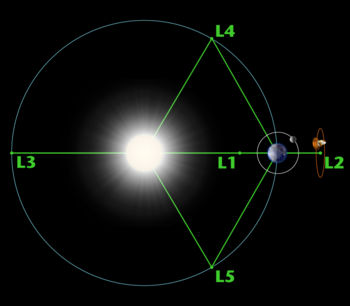
| + | [[Image:Lagrange_A.jpg|thumb|350px|A diagram showing the five Lagrangian points in a two-body system with one body far more massive than the other (e.g. the Sun and the Earth). In such a system L<sub>3</sub>–L<sub>5</sub> will appear to share the secondary's orbit, although in fact they are situated slightly outside it.]] | ||
The five Lagrangian points are labeled and defined as follows: | The five Lagrangian points are labeled and defined as follows: | ||
| − | ==L<sub>1</sub>== | + | ===L<sub>1</sub>=== |
| − | The '''L<sub>1</sub>''' point lies on the line defined by the two large masses M<sub>1</sub> and M<sub>2</sub>, and between them. | + | The '''L<sub>1</sub>''' point lies on the line defined by the two large masses M<sub>1</sub> and M<sub>2</sub>, and between them. It is the most intuitively understood of the Lagrangian points: the one where the gravitational attractions of the two other objects effectively cancel each other out. |
| − | '''Example:''' An object which [[orbit]]s the [[Sun]] more closely than the [[Earth]] would normally have a shorter orbital period than the Earth, but that ignores the effect of the Earth's own gravitational pull. If the object is directly between the Earth and the Sun, then the effect of the Earth's gravity is to weaken the force pulling the object towards the Sun, and therefore increase the orbital period of the object. The closer to Earth the object is, the greater this effect is. At the L<sub>1</sub> point, the orbital period of the object becomes exactly equal to the Earth's orbital period. | + | :'''Example:''' An object which [[orbit]]s the [[Sun]] more closely than the [[Earth]] would normally have a shorter orbital period than the Earth, but that ignores the effect of the Earth's own gravitational pull. If the object is directly between the Earth and the Sun, then the effect of the Earth's gravity is to weaken the force pulling the object towards the Sun, and therefore increase the orbital period of the object. The closer to Earth the object is, the greater this effect is. At the L<sub>1</sub> point, the orbital period of the object becomes exactly equal to the Earth's orbital period. |
| − | The Sun | + | The Sun–Earth L<sub>1</sub> is ideal for making observations of the Sun. Objects here are never shadowed by the Earth or the Moon. The [[Solar and Heliospheric Observatory]] (SOHO) is stationed in a [[Lagrangian point#Stability|Halo orbit]] at the L<sub>1</sub> and the [[Advanced Composition Explorer]] (ACE) is in a [[Lissajous]] orbit, also at the L<sub>1</sub> point. The Earth–Moon L<sub>1</sub> allows easy access to lunar and earth orbits with minimal [[delta-v]], and would be ideal for a half-way manned space station intended to help transport cargo and personnel to the Moon and back. |
| − | ==L<sub>2</sub>== | + | ===L<sub>2</sub>=== |
| − | The '''L<sub>2</sub>''' point lies on the line defined by the two large masses, | + | The '''L<sub>2</sub>''' point lies on the line defined by the two large masses, beyond the smaller of the two. |
| − | '''Example:''' On the | + | :'''Example:''' On the side of the Earth away from the Sun, the orbital period of an object would normally be greater than that of the Earth. The extra pull of the Earth's gravity decreases the orbital period of the object, and at the L<sub>2</sub> point that orbital period becomes equal to the Earth's. |
| − | Sun | + | Sun–Earth L<sub>2</sub> is a good spot for space-based observatories. Because an object around L<sub>2</sub> will maintain the same orientation with respect to the Sun and Earth, shielding and calibration are much simpler. The [[Wilkinson Microwave Anisotropy Probe]] is already in orbit around the Sun–Earth L<sub>2</sub>. The future [[Herschel Space Observatory]] and [[Gaia probe]] as well as the proposed [[James Webb Space Telescope]] will be placed at the Sun–Earth L<sub>2</sub>. Earth–Moon L<sub>2</sub> would be a good location for a [[communications satellite]] covering the Moon's far side. |
| − | If M<sub>2</sub> is much smaller than M<sub>1</sub> then L<sub>1</sub> and L<sub>2</sub> are at approximately equal distances ''r'' from M<sub>2</sub>, equal to the radius of the [[Hill sphere]], given by: | + | If M<sub>2</sub> is much smaller than M<sub>1</sub>, then L<sub>1</sub> and L<sub>2</sub> are at approximately equal distances ''r'' from M<sub>2</sub>, equal to the radius of the [[Hill sphere]], given by: |
:<math>r \approx R \sqrt[3]{\frac{M_2}{3 M_1}}</math> | :<math>r \approx R \sqrt[3]{\frac{M_2}{3 M_1}}</math> | ||
| − | where ''R'' is the distance between the two bodies | + | where ''R'' is the distance between the two bodies. |
| − | This distance can be described as being such that the [[orbital period]] corresponding to a circular orbit with this distance as radius around M<sub>2</sub> in the absence of M<sub>1</sub>, is that of M<sub>2</sub> around M<sub>1</sub>, divided by <math>\sqrt{3}\approx 1.73</math>. | + | This distance can be described as being such that the [[orbital period]], corresponding to a circular orbit with this distance as radius around M<sub>2</sub> in the absence of M<sub>1</sub>, is that of M<sub>2</sub> around M<sub>1</sub>, divided by <math>\sqrt{3}\approx 1.73</math>. |
Examples: | Examples: | ||
| Line 35: | Line 46: | ||
*Earth and [[Moon]]: 61,500 km from the Moon | *Earth and [[Moon]]: 61,500 km from the Moon | ||
| − | ==L<sub>3</sub>== | + | ===L<sub>3</sub>=== |
| − | The '''L<sub>3</sub>''' point lies on the line defined by the two large masses, | + | The '''L<sub>3</sub>''' point lies on the line defined by the two large masses, beyond the larger of the two. |
| − | '''Example:''' | + | :'''Example:''' L<sub>3</sub> in the Sun–Earth system exists on the opposite side of the Sun, a little outside the Earth's orbit but slightly closer to the Sun than the Earth is.<ref>This apparent contradiction is because the Sun is also affected by the Earth's gravity, and so orbits around the two bodies' [[barycentre]], which is however well inside the body of the Sun.</ref> Here, the combined pull of the Earth and Sun again causes the object to orbit with the same period as the Earth. The Sun–Earth L<sub>3</sub> point was a popular place to put a "[[Counter-Earth]]" in pulp [[science fiction]] and [[comic book]]s - though of course, once space based observation was possible via satellites and probes, it was shown to hold no such object. In actual fact, Sun–Earth L<sub>3</sub> is highly unstable, because the gravitational forces of the other planets outweigh that of the Earth ([[Venus]], for example, comes within 0.3 [[Astronomical unit|AU]] of L<sub>3</sub> every 20 months). |
| − | |||
| − | ==L<sub>4</sub> and L<sub>5</sub>== | + | ===L<sub>4</sub> and L<sub>5</sub>=== |
| − | The '''L<sub>4</sub>''' and '''L<sub>5</sub>''' points lie at the third | + | [[Image:L4 diagram.svg|thumb|right|200px|Gravitational accelerations at L<sub>4</sub>]] |
| + | The '''L<sub>4</sub>''' and '''L<sub>5</sub>''' points lie at the third corners of the two [[equilateral triangle]]s in the plane of orbit whose common base is the line between the centres of the two masses, such that the point is ahead of (L<sub>4</sub>), or behind (L<sub>5</sub>), the smaller mass with regard to its orbit around the larger mass. | ||
| − | L<sub>4</sub> and L<sub>5</sub> are | + | The reason these points are in balance is that at L<sub>4</sub> and L<sub>5</sub>, the distances to the two masses are equal. Accordingly, the gravitational forces from the two massive bodies are in the same ratio as the masses of the two bodies, and so the resultant force acts through the [[barycentre]] of the system; additionally, the geometry of the triangle ensures that the [[resultant]] acceleration is to the distance from the barycentre in the same [[ratio]] as for the two massive bodies. The barycentre being both the [[centre of mass]] and centre of rotation of the system, this resultant force is exactly that required to keep a body at the Lagrange point in orbital [[Dynamic equilibrium|equilibrium]] with the rest of the system. (Indeed, the third body need not have negligible mass; the general triangular configuration was discovered by Lagrange in work on the [[3-body problem]].) |
| − | ''' | + | L<sub>4</sub> and L<sub>5</sub> are sometimes called ''triangular Lagrange points'' or ''Trojan points''. The name ''Trojan points'' comes from the [[Trojan asteroids]] at the Sun-[[Jupiter (planet)|Jupiter]] L<sub>4</sub> and L<sub>5</sub> points, which themselves are named after characters from [[Homer]]'s [[Iliad]] (the legendary siege of [[Troy]]). Asteroids at the L4 point, which leads Jupiter are referred to as the '[[List of Trojan asteroids (Greek camp)|Greek camp]]' while at the L5 point they are referred to as the '[[List of Trojan asteroids (Trojan camp)|Trojan camp]]', and asteroids there are (largely) named after characters from the respective sides of the war. |
| + | |||
| + | :'''Examples:'''<br> | ||
| + | * The Sun–Earth L<sub>4</sub> and L<sub>5</sub> points lie 60° ahead of and 60° behind the Earth as it orbits the Sun. They contain interplanetary dust. | ||
| + | * The Earth–Moon L<sub>4</sub> and L<sub>5</sub> points lie 60° ahead of and 60° behind the Moon as it orbits the Earth. They also contain interplanetary dust in what is called [[Kordylewski cloud]]. | ||
| + | * The Sun–Jupiter L<sub>4</sub> and L<sub>5</sub> points are occupied by the [[Trojan asteroid]]s. | ||
| + | * [[Neptune]] has Trojan [[Kuiper Belt Object]]s at its L<sub>4</sub> and L<sub>5</sub> points. | ||
| + | * [[Saturn]]'s moon [[Tethys (moon)|Tethys]] has two much smaller satellites at its L<sub>4</sub> and L<sub>5</sub> points named [[Telesto (moon)|Telesto]] and [[Calypso (moon)|Calypso]], respectively. | ||
| + | * Saturn's moon [[Dione (moon)|Dione]] has smaller moons [[Helene (moon)|Helene]] and [[Polydeuces (moon)|Polydeuces]] at its L<sub>4</sub> and L<sub>5</sub> points, respectively. | ||
| + | * The [[giant impact hypothesis]] suggests that an object named [[Theia (planet)|Theia]] formed at L<sub>4</sub> or L<sub>5</sub> and crashed into the Earth after its orbit destabilized, forming the moon. | ||
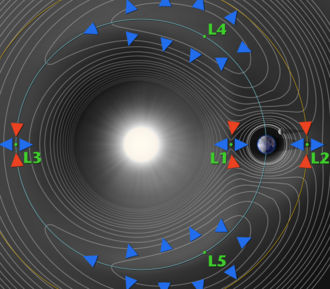
==Stability== | ==Stability== | ||
| − | The first three Lagrangian points are stable only in the plane | + | The first three Lagrangian points are technically stable only in the plane perpendicular to the line between the two bodies. This can be seen most easily by considering the L<sub>1</sub> point. A test mass displaced perpendicularly from the central line would feel a force pulling it back towards the equilibrium point. This is because the lateral components of the two masses' gravity would add to produce this force, whereas the components along the axis between them would balance out. However, if an object located at the L<sub>1</sub> point drifted closer to one of the masses, the gravitational attraction it felt from that mass would be greater, and it would be pulled closer. (The pattern is very similar to that of {{exd|tidal force|tidal forces}}.) |
| + | |||
| + | Although the L<sub>1</sub>, L<sub>2</sub>, and L<sub>3</sub> points are nominally unstable, it turns out that it is possible to find stable periodic orbits around these points, at least in the restricted three-body problem. These perfectly periodic orbits, referred to as "halo" orbits, do not exist in a full n-body dynamical system such as the {{exd|Solar System|Solar system}}. However, quasi-periodic (i.e. bounded but not precisely repeating) orbits following Lissajous curve trajectories do exist in the n-body system. These quasi-periodic {{exd|Lissajous orbit|Lissajous orbits}} are what all Lagrangian point missions to date have used. Although they are not perfectly stable, a relatively modest effort at {{exd|Station Keeping|station keeping}} can allow a spacecraft to stay in a desired Lissajous orbit for an extended period of time. It also turns out that, at least in the case of Sun–Earth L<sub>1</sub> missions, it is actually preferable to place the spacecraft in a large amplitude (100,000–200,000 km) Lissajous orbit instead of having it sit at the Lagrangian point, because this keeps the spacecraft off the direct Sun–Earth line and thereby reduces the impacts of solar interference on the Earth–spacecraft communications links. Another interesting and useful property of the collinear Lagrangian points and their associated Lissajous orbits is that they serve as "gateways" to control the chaotic trajectories of the [[Interplanetary Transport Network]]. | ||
| + | |||
| + | In contrast to the collinear Lagrangian points, the triangular points (L<sub>4</sub> and L<sub>5</sub>) are stable equilibria (cf. attractor), provided the ratio of M<sub>1</sub>/M<sub>2</sub> is greater than 24.96<ref>Actually <math>\left(25\left(\frac{1-\sqrt{1-4/625}}{2}\right)\right)^{-1}</math></ref><ref name="cornish">[http://map.gsfc.nasa.gov/ContentMedia/lagrange.pdf The Lagrange Points] – Neil J. Cornish with input from Jeremy Goodman</ref>. This is the case for the Sun/Earth and, by a smaller margin, the Earth/Moon systems. When a body at these points is perturbed, it moves away from the point, but the {{exd|Coriolis Force|Coriolis effect}} then acts, and bends the object's path into a stable, kidney bean‐shaped orbit around the point (as seen in the rotating frame of reference). However, in the Earth-Moon case the problem of stability is greatly complicated by the appreciable solar gravitational influence.<ref> | ||
| + | http://www.rfreitas.com/Astro/SearchIcarus1980.htm A Search for Natural or Artificial Objects Located at the Earth-Moon Libration Points; Icarus.</ref> | ||
| − | + | ==Lagrangian point missions== | |
| − | + | The Lagrangian point orbits have unique characteristics that have made them a good choice for performing some kinds of missions. [[NASA]] has operated a number of spacecraft in orbit around the Sun-Earth L<sub>1</sub> and L<sub>2</sub> points, including | |
| + | {| style="border:1px solid black" | ||
| + | |- style="background:#BFBFBF" | ||
| + | | Mission || Lagrangian point | ||
| + | |- | ||
| + | | [[Advanced Composition Explorer|Advanced Composition Explorer (ACE)]] || <div style="text-align:center;">L<sub>1</sub></div> | ||
| + | |- style="background:#EFEFEF" | ||
| + | | [[Genesis (spacecraft)|Genesis]] || <div style="text-align:center;">L<sub>1</sub></div> | ||
| + | |- | ||
| + | | [[International Cometary Explorer|International Sun/Earth Explorer 3 (ISEE-3)]] || <div style="text-align:center;">L<sub>1</sub></div> | ||
| + | |- style="background:#EFEFEF" | ||
| + | | [[Solar and Heliospheric Observatory|Solar and Heliospheric Observatory (SOHO)]] || <div style="text-align:center;">L<sub>1</sub></div> | ||
| + | |- | ||
| + | | [[WMAP|Wilkinson Microwave Anisotropy Probe (WMAP)]] || <div style="text-align:center;">L<sub>2</sub></div> | ||
| + | |} | ||
| − | + | The [[James Webb Space Telescope]] is also planned to be placed in orbit around L<sub>2</sub>. | |
| − | + | The [[L5 Society]] was a precursor of the [[National Space Society]], and promoted the possibility of establishing a colony and manufacturing facility in orbit around the L<sub>4</sub> or L<sub>5</sub> points in the Earth–Moon system. | |
| − | + | ==Natural examples== | |
| − | + | In the Sun–[[Jupiter (planet)|Jupiter]] system several thousand [[asteroid]]s, collectively referred to as [[Trojan asteroid]]s, are in orbits around the Sun–Jupiter L<sub>4</sub> and L<sub>5</sub> points. Other bodies can be found in the Sun–[[Saturn (planet)|Saturn]], Sun–[[Mars (planet)|Mars]], Sun-[[Neptune (planet)|Neptune]], Jupiter–Jovian [[satellite]], and Saturn-Saturnian satellite systems. There are no known large bodies in the Sun–Earth system's Trojan points, but clouds of dust surrounding the L<sub>4</sub> and L<sub>5</sub> points were discovered in the [[1950s]]. Clouds of dust, called [[Kordylewski cloud]]s, even fainter than the notoriously weak [[gegenschein]], are also present in the L<sub>4</sub> and L<sub>5</sub> of the Earth–[[Moon]] system. | |
| − | + | The Saturnian moon [[Tethys (moon)|Tethys]] has two smaller moons in its L<sub>4</sub> and L<sub>5</sub> points, [[Telesto (moon)|Telesto]] and [[Calypso (moon)|Calypso]]. The Saturnian moon [[Dione (moon)|Dione]] also has two Lagrangian co-orbitals, [[Helene (moon)|Helene]] at its L<sub>4</sub> point and [[Polydeuces (moon)|Polydeuces]] at L<sub>5</sub>. The moons wander [[azimuth]]ally about the Lagrangian points, with Polydeuces describing the largest deviations, moving up to 32 degrees away from the Saturn–Dione L<sub>5</sub> point. Tethys and Dione are hundreds of times more massive than their "escorts" (see the moons' articles for exact diameter figures; masses are not known in several cases), and Saturn is far more massive still, which makes the overall system stable. | |
| − | The Earth's companion object [[3753 Cruithne]] is in a somewhat Trojan-like | + | ===Other co-orbitals=== |
| + | |||
| + | The Earth's companion object [[3753 Cruithne]] is in a relationship with the Earth which is somewhat Trojan-like, but different from a true Trojan. This asteroid occupies one of two regular solar orbits, one of them slightly smaller and faster than the Earth's orbit, and the other slightly larger and slower. The asteroid periodically alternates between these two orbits due to close encounters with Earth. When the asteroid is in the smaller, faster orbit and approaches the Earth, it loses orbital energy to the Earth and moves into the larger, slower orbit. It then falls farther and farther behind the earth, and eventually Earth approaches it from the other direction. Then the asteroid gains orbital energy from the Earth, and the asteroid moves back into the smaller orbit, thus beginning the cycle anew. The cycle has no noticeable impact on the length of the year, because Earth's mass is over 20 [[1000000000 (number)|billion]] (2 × 10<sup>10</sup>) times more than 3753 Cruithne. | ||
| + | |||
| + | [[Epimetheus (moon)|Epimetheus]] and [[Janus (moon)|Janus]], satellites of Saturn, have a similar relationship, though they are of similar masses and so actually exchange orbits with each other periodically. (Janus is roughly 4 times more massive, but still light enough for its orbit to be altered.) Another similar configuration is known as [[orbital resonance]], in which orbiting bodies tend to have periods of a simple integer [[ratio]], due to their interaction. | ||
| + | |||
| + | |||
| + | |||
| + | ==Notes and references== | ||
| + | <references/> | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==External links== | ==External links== | ||
| − | *[http://www.physics.montana.edu/faculty/cornish/lagrange.html Explanation of Lagrange points | + | *[http://www.physics.montana.edu/faculty/cornish/lagrange.html Explanation of Lagrange points] – Prof. Neil J. Cornish |
| − | *[http://math.ucr.edu/home/baez/lagrange.html Explanation of Lagrange points | + | *[http://map.gsfc.nasa.gov/m_mm/ob_techorbit1.html A NASA explanation] - also attributed to Neil J. Cornish |
| − | *[http:// | + | *[http://math.ucr.edu/home/baez/lagrange.html Explanation of Lagrange points] – Prof. John Baez |
| + | *[http://www.merlyn.demon.co.uk/gravity4.htm Geometry and calculations of Lagrange points] – Dr J R Stockton | ||
| + | *[http://www.phy6.org/stargaze/Slagrang.htm Locations of Lagrange points, with approximations] – Dr. David P. Stern | ||
| + | <!-- Excluded {{orbits}} as it called {{Navbox_generic}} which called 17 more templates --> | ||
| + | |||
| + | [[Category:Wikipedia Based Articles]] | ||
[[Category:Celestial mechanics]] | [[Category:Celestial mechanics]] | ||
| − | + | [[Category:Earth orbits]] | |
| − | [[ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 16:42, 19 June 2007
This article is tagged for relocation to the Exoplatz.
| Work on this article has outpaced copyediting on it. You can help Lunarpedia by formatting, editing, or tidying it. |
The Lagrangian points; also Lagrange point, L-point, or libration point), are the five positions in an orbital configuration where a small object affected only by gravity can theoretically be stationary relative to two larger objects (such as a satellite with respect to the Earth and Moon). The Lagrange points mark positions where the combined gravitational pull of the two large masses provides precisely the centripetal force required to rotate with them. They are analogous to geosynchronous orbits in that they allow an object to be in a "fixed" position in space rather than an orbit in which its relative position changes continuously.
A more precise but technical definition is that the Lagrangian points are the stationary solutions of the circular restricted three-body problem. For example, given two massive bodies in circular orbits around their common center of mass, there are five positions in space where a third body, of comparatively negligible mass, could be placed which would then maintain its position relative to the two massive bodies. As seen in a rotating reference frame with the same period as the two co-orbiting bodies, the gravitational fields of two massive bodies combined with the centrifugal force are in balance at the Lagrangian points, allowing the third body to be stationary with respect to the first two bodies.
Contents
History and concepts
In 1772, the famed Italian-French mathematician Joseph-Louis Lagrange![]() was working on the famous three-body problem
was working on the famous three-body problem![]() when he discovered an interesting quirk in the results. Originally, he had set out to discover a way to easily calculate the gravitational interaction between arbitrary numbers of bodies in a system, because Newtonian mechanics conclude that such a system results in the bodies orbiting chaotically until there is a collision, or a body is thrown out of the system so that equilibrium can be achieved. The logic behind this conclusion is that a system with one body is trivial, as it is merely static relative to itself; a system with two bodies is very simple to solve for, as the bodies orbit around their common center of gravity. However, once more than two bodies are introduced, the mathematical calculations become very complicated. A situation arises where you would have to calculate every gravitational interaction between every object at every point along its trajectory.
when he discovered an interesting quirk in the results. Originally, he had set out to discover a way to easily calculate the gravitational interaction between arbitrary numbers of bodies in a system, because Newtonian mechanics conclude that such a system results in the bodies orbiting chaotically until there is a collision, or a body is thrown out of the system so that equilibrium can be achieved. The logic behind this conclusion is that a system with one body is trivial, as it is merely static relative to itself; a system with two bodies is very simple to solve for, as the bodies orbit around their common center of gravity. However, once more than two bodies are introduced, the mathematical calculations become very complicated. A situation arises where you would have to calculate every gravitational interaction between every object at every point along its trajectory.
Lagrange, however, wanted to make this simpler. He did so with a simple conclusion: The trajectory of an object is determined by finding a path that minimizes the action over time. This is found by subtracting the potential energy from the kinetic energy. With this way of thinking, Lagrange re-formulated the classical Newtonian mechanics to give rise to Lagrangian mechanics. With his new system of calculations, Lagrange’s work led him to hypothesize how a third body of negligible mass would orbit around two larger bodies which were already in a near-circular orbit. In a frame of reference that rotates with the larger bodies, he found five specific fixed points where the third body experiences zero net force as it follows the circular orbit of its host bodies (planets).[1] These points were named “Lagrangian points” in Lagrange's honor. It took over a hundred years before his mathematical theory was observed with the discovery of the Trojan asteroids in the 1900s at the Lagrange points of the Sun-Jupiter system.
In the more general case of elliptical orbits, there are no longer stationary points in the same sense: it becomes more of a Lagrangian “area”. The Lagrangian points constructed at each point in time as in the circular case form stationary elliptical orbits which are similar to the orbits of the massive bodies. This is due to Newton's second law (<math>d\mathbf{p}/dt=\mathbf{F}</math>), where p = mv (p the momentum, m the mass, and v the velocity) is invariant if force and position are scaled by the same factor. A body at a Lagrangian point orbits with the same period as the two massive bodies in the circular case, implying that it has the same ratio of gravitational force to radial distance as they do. This fact is independent of the circularity of the orbits, and it implies that the elliptical orbits traced by the Lagrangian points are solutions of the equation of motion of the third body.
The Lagrangian points
The five Lagrangian points are labeled and defined as follows:
L1
The L1 point lies on the line defined by the two large masses M1 and M2, and between them. It is the most intuitively understood of the Lagrangian points: the one where the gravitational attractions of the two other objects effectively cancel each other out.
- Example: An object which orbits the Sun more closely than the Earth would normally have a shorter orbital period than the Earth, but that ignores the effect of the Earth's own gravitational pull. If the object is directly between the Earth and the Sun, then the effect of the Earth's gravity is to weaken the force pulling the object towards the Sun, and therefore increase the orbital period of the object. The closer to Earth the object is, the greater this effect is. At the L1 point, the orbital period of the object becomes exactly equal to the Earth's orbital period.
The Sun–Earth L1 is ideal for making observations of the Sun. Objects here are never shadowed by the Earth or the Moon. The Solar and Heliospheric Observatory (SOHO) is stationed in a Halo orbit at the L1 and the Advanced Composition Explorer (ACE) is in a Lissajous orbit, also at the L1 point. The Earth–Moon L1 allows easy access to lunar and earth orbits with minimal delta-v, and would be ideal for a half-way manned space station intended to help transport cargo and personnel to the Moon and back.
L2
The L2 point lies on the line defined by the two large masses, beyond the smaller of the two.
- Example: On the side of the Earth away from the Sun, the orbital period of an object would normally be greater than that of the Earth. The extra pull of the Earth's gravity decreases the orbital period of the object, and at the L2 point that orbital period becomes equal to the Earth's.
Sun–Earth L2 is a good spot for space-based observatories. Because an object around L2 will maintain the same orientation with respect to the Sun and Earth, shielding and calibration are much simpler. The Wilkinson Microwave Anisotropy Probe is already in orbit around the Sun–Earth L2. The future Herschel Space Observatory and Gaia probe as well as the proposed James Webb Space Telescope will be placed at the Sun–Earth L2. Earth–Moon L2 would be a good location for a communications satellite covering the Moon's far side.
If M2 is much smaller than M1, then L1 and L2 are at approximately equal distances r from M2, equal to the radius of the Hill sphere, given by:
- <math>r \approx R \sqrt[3]{\frac{M_2}{3 M_1}}</math>
where R is the distance between the two bodies.
This distance can be described as being such that the orbital period, corresponding to a circular orbit with this distance as radius around M2 in the absence of M1, is that of M2 around M1, divided by <math>\sqrt{3}\approx 1.73</math>.
Examples:
L3
The L3 point lies on the line defined by the two large masses, beyond the larger of the two.
- Example: L3 in the Sun–Earth system exists on the opposite side of the Sun, a little outside the Earth's orbit but slightly closer to the Sun than the Earth is.[2] Here, the combined pull of the Earth and Sun again causes the object to orbit with the same period as the Earth. The Sun–Earth L3 point was a popular place to put a "Counter-Earth" in pulp science fiction and comic books - though of course, once space based observation was possible via satellites and probes, it was shown to hold no such object. In actual fact, Sun–Earth L3 is highly unstable, because the gravitational forces of the other planets outweigh that of the Earth (Venus, for example, comes within 0.3 AU of L3 every 20 months).
L4 and L5
The L4 and L5 points lie at the third corners of the two equilateral triangles in the plane of orbit whose common base is the line between the centres of the two masses, such that the point is ahead of (L4), or behind (L5), the smaller mass with regard to its orbit around the larger mass.
The reason these points are in balance is that at L4 and L5, the distances to the two masses are equal. Accordingly, the gravitational forces from the two massive bodies are in the same ratio as the masses of the two bodies, and so the resultant force acts through the barycentre of the system; additionally, the geometry of the triangle ensures that the resultant acceleration is to the distance from the barycentre in the same ratio as for the two massive bodies. The barycentre being both the centre of mass and centre of rotation of the system, this resultant force is exactly that required to keep a body at the Lagrange point in orbital equilibrium with the rest of the system. (Indeed, the third body need not have negligible mass; the general triangular configuration was discovered by Lagrange in work on the 3-body problem.)
L4 and L5 are sometimes called triangular Lagrange points or Trojan points. The name Trojan points comes from the Trojan asteroids at the Sun-Jupiter L4 and L5 points, which themselves are named after characters from Homer's Iliad (the legendary siege of Troy). Asteroids at the L4 point, which leads Jupiter are referred to as the 'Greek camp' while at the L5 point they are referred to as the 'Trojan camp', and asteroids there are (largely) named after characters from the respective sides of the war.
- Examples:
- The Sun–Earth L4 and L5 points lie 60° ahead of and 60° behind the Earth as it orbits the Sun. They contain interplanetary dust.
- The Earth–Moon L4 and L5 points lie 60° ahead of and 60° behind the Moon as it orbits the Earth. They also contain interplanetary dust in what is called Kordylewski cloud.
- The Sun–Jupiter L4 and L5 points are occupied by the Trojan asteroids.
- Neptune has Trojan Kuiper Belt Objects at its L4 and L5 points.
- Saturn's moon Tethys has two much smaller satellites at its L4 and L5 points named Telesto and Calypso, respectively.
- Saturn's moon Dione has smaller moons Helene and Polydeuces at its L4 and L5 points, respectively.
- The giant impact hypothesis suggests that an object named Theia formed at L4 or L5 and crashed into the Earth after its orbit destabilized, forming the moon.
Stability
The first three Lagrangian points are technically stable only in the plane perpendicular to the line between the two bodies. This can be seen most easily by considering the L1 point. A test mass displaced perpendicularly from the central line would feel a force pulling it back towards the equilibrium point. This is because the lateral components of the two masses' gravity would add to produce this force, whereas the components along the axis between them would balance out. However, if an object located at the L1 point drifted closer to one of the masses, the gravitational attraction it felt from that mass would be greater, and it would be pulled closer. (The pattern is very similar to that of tidal forces![]() .)
.)
Although the L1, L2, and L3 points are nominally unstable, it turns out that it is possible to find stable periodic orbits around these points, at least in the restricted three-body problem. These perfectly periodic orbits, referred to as "halo" orbits, do not exist in a full n-body dynamical system such as the Solar system![]() . However, quasi-periodic (i.e. bounded but not precisely repeating) orbits following Lissajous curve trajectories do exist in the n-body system. These quasi-periodic Lissajous orbits
. However, quasi-periodic (i.e. bounded but not precisely repeating) orbits following Lissajous curve trajectories do exist in the n-body system. These quasi-periodic Lissajous orbits![]() are what all Lagrangian point missions to date have used. Although they are not perfectly stable, a relatively modest effort at station keeping
are what all Lagrangian point missions to date have used. Although they are not perfectly stable, a relatively modest effort at station keeping![]() can allow a spacecraft to stay in a desired Lissajous orbit for an extended period of time. It also turns out that, at least in the case of Sun–Earth L1 missions, it is actually preferable to place the spacecraft in a large amplitude (100,000–200,000 km) Lissajous orbit instead of having it sit at the Lagrangian point, because this keeps the spacecraft off the direct Sun–Earth line and thereby reduces the impacts of solar interference on the Earth–spacecraft communications links. Another interesting and useful property of the collinear Lagrangian points and their associated Lissajous orbits is that they serve as "gateways" to control the chaotic trajectories of the Interplanetary Transport Network.
can allow a spacecraft to stay in a desired Lissajous orbit for an extended period of time. It also turns out that, at least in the case of Sun–Earth L1 missions, it is actually preferable to place the spacecraft in a large amplitude (100,000–200,000 km) Lissajous orbit instead of having it sit at the Lagrangian point, because this keeps the spacecraft off the direct Sun–Earth line and thereby reduces the impacts of solar interference on the Earth–spacecraft communications links. Another interesting and useful property of the collinear Lagrangian points and their associated Lissajous orbits is that they serve as "gateways" to control the chaotic trajectories of the Interplanetary Transport Network.
In contrast to the collinear Lagrangian points, the triangular points (L4 and L5) are stable equilibria (cf. attractor), provided the ratio of M1/M2 is greater than 24.96[3][4]. This is the case for the Sun/Earth and, by a smaller margin, the Earth/Moon systems. When a body at these points is perturbed, it moves away from the point, but the Coriolis effect![]() then acts, and bends the object's path into a stable, kidney bean‐shaped orbit around the point (as seen in the rotating frame of reference). However, in the Earth-Moon case the problem of stability is greatly complicated by the appreciable solar gravitational influence.[5]
then acts, and bends the object's path into a stable, kidney bean‐shaped orbit around the point (as seen in the rotating frame of reference). However, in the Earth-Moon case the problem of stability is greatly complicated by the appreciable solar gravitational influence.[5]
Lagrangian point missions
The Lagrangian point orbits have unique characteristics that have made them a good choice for performing some kinds of missions. NASA has operated a number of spacecraft in orbit around the Sun-Earth L1 and L2 points, including
| Mission | Lagrangian point |
| Advanced Composition Explorer (ACE) | L1
|
| Genesis | L1
|
| International Sun/Earth Explorer 3 (ISEE-3) | L1
|
| Solar and Heliospheric Observatory (SOHO) | L1
|
| Wilkinson Microwave Anisotropy Probe (WMAP) | L2
|
The James Webb Space Telescope is also planned to be placed in orbit around L2.
The L5 Society was a precursor of the National Space Society, and promoted the possibility of establishing a colony and manufacturing facility in orbit around the L4 or L5 points in the Earth–Moon system.
Natural examples
In the Sun–Jupiter system several thousand asteroids, collectively referred to as Trojan asteroids, are in orbits around the Sun–Jupiter L4 and L5 points. Other bodies can be found in the Sun–Saturn, Sun–Mars, Sun-Neptune, Jupiter–Jovian satellite, and Saturn-Saturnian satellite systems. There are no known large bodies in the Sun–Earth system's Trojan points, but clouds of dust surrounding the L4 and L5 points were discovered in the 1950s. Clouds of dust, called Kordylewski clouds, even fainter than the notoriously weak gegenschein, are also present in the L4 and L5 of the Earth–Moon system.
The Saturnian moon Tethys has two smaller moons in its L4 and L5 points, Telesto and Calypso. The Saturnian moon Dione also has two Lagrangian co-orbitals, Helene at its L4 point and Polydeuces at L5. The moons wander azimuthally about the Lagrangian points, with Polydeuces describing the largest deviations, moving up to 32 degrees away from the Saturn–Dione L5 point. Tethys and Dione are hundreds of times more massive than their "escorts" (see the moons' articles for exact diameter figures; masses are not known in several cases), and Saturn is far more massive still, which makes the overall system stable.
Other co-orbitals
The Earth's companion object 3753 Cruithne is in a relationship with the Earth which is somewhat Trojan-like, but different from a true Trojan. This asteroid occupies one of two regular solar orbits, one of them slightly smaller and faster than the Earth's orbit, and the other slightly larger and slower. The asteroid periodically alternates between these two orbits due to close encounters with Earth. When the asteroid is in the smaller, faster orbit and approaches the Earth, it loses orbital energy to the Earth and moves into the larger, slower orbit. It then falls farther and farther behind the earth, and eventually Earth approaches it from the other direction. Then the asteroid gains orbital energy from the Earth, and the asteroid moves back into the smaller orbit, thus beginning the cycle anew. The cycle has no noticeable impact on the length of the year, because Earth's mass is over 20 billion (2 × 1010) times more than 3753 Cruithne.
Epimetheus and Janus, satellites of Saturn, have a similar relationship, though they are of similar masses and so actually exchange orbits with each other periodically. (Janus is roughly 4 times more massive, but still light enough for its orbit to be altered.) Another similar configuration is known as orbital resonance, in which orbiting bodies tend to have periods of a simple integer ratio, due to their interaction.
Notes and references
- ↑ Joseph-Louis Lagrange - Oeuvres de Lagrange, 1867-1892; Gauthier-Villars. Tome 6, Chapitre II: Essai sur le problème des trois corps, 272-292, http://gallica.bnf.fr/Catalogue/noticesInd/FRBNF30719104.htm
- ↑ This apparent contradiction is because the Sun is also affected by the Earth's gravity, and so orbits around the two bodies' barycentre, which is however well inside the body of the Sun.
- ↑ Actually <math>\left(25\left(\frac{1-\sqrt{1-4/625}}{2}\right)\right)^{-1}</math>
- ↑ The Lagrange Points – Neil J. Cornish with input from Jeremy Goodman
- ↑ http://www.rfreitas.com/Astro/SearchIcarus1980.htm A Search for Natural or Artificial Objects Located at the Earth-Moon Libration Points; Icarus.
External links
- Explanation of Lagrange points – Prof. Neil J. Cornish
- A NASA explanation - also attributed to Neil J. Cornish
- Explanation of Lagrange points – Prof. John Baez
- Geometry and calculations of Lagrange points – Dr J R Stockton
- Locations of Lagrange points, with approximations – Dr. David P. Stern